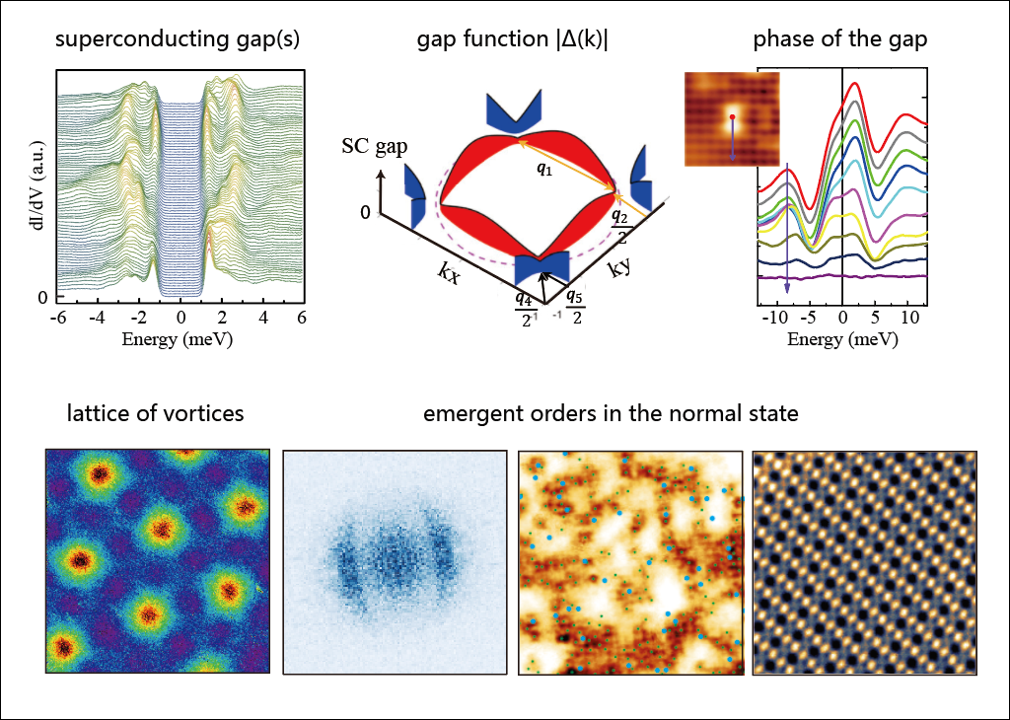
The Bardeen-Cooper-Schrieffer (BCS) theory established in 1957 have gained great success as the first microscopic explanation of superconductivity. However, in the following decades people discovered numerous systems in which the superconducting states exhibit behaviors that deviate from the description of the BCS theory, such as Cu- and Fe-based high temperature superconductors, heavy Fermions, organic superconductors etc. These superconducting states are generally recognized to be 'unconventional'; comparing with the ordinary BCS superconductors, they usually break additional symmetries, thus are manifested by novel gap functions and physical properties. Furthermore, in many of these interesting systems, the electron-electron interactions cannot be swept under the carpet. The normal states in which the superconductivity sets in also host mysterious emergent phenomena that cannot be understood within the frame of Landau-Fermi-liquid theory. With STM/S, we have studied single-layer strontium ruthenate, Fe-based superconductors, iridates and kagome superconductor.

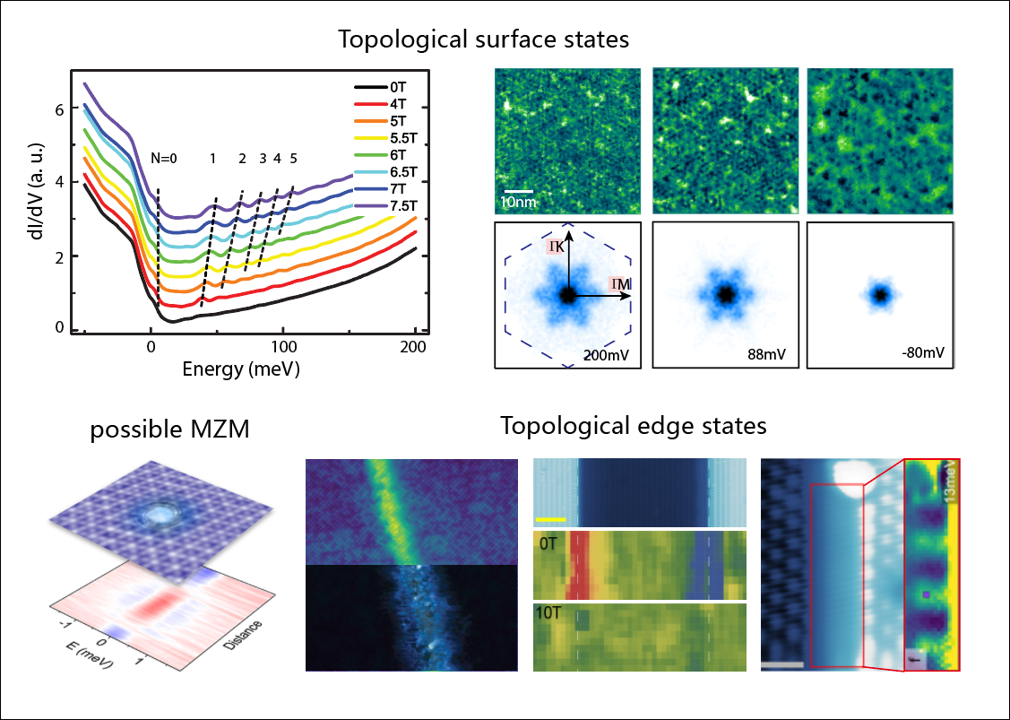
Topology is a geometric property that cannot be changed by any smooth deformations. Similarly, the electronic property of a topological material is also robust and resists disruptions: the electronic band structure belongs to a different topological class from that of ordinary insulators and metals. At the boundary of the material, there can be topologically protected edge states with unique electronic properties, such as spin-momentum locking, being zero mass, etc. Introducing many-body interactions, e.g. superconducting pairing or magnetic interactions into topological compounds could result in new quantum phase of matter, in which  quantum anomalous Hall effect and Majorana zero/edge modes may be realized. We have worked on 3D topological insulator(TI) (Bi, Sb)₂Te₃, intrinsic antiferromagnetic TI MnBi₂Te₄, Weyl semimetal MoTe₂ and topological superconductor candidates Sr₂RuO₄, UTe₂, Fe(Se, Te) and CsV₃Sb₅.